The majority of mathematical studies have no practical use. There aren’t many places where knot theory doesn’t stand out as an anomaly. Knots are a common occurrence in the world around us, whether we’re dealing with woven materials, shoe laces, or mountaineering ropes. Knots were in common use among humans 500,000 years ago. There is evidence to suggest that several species of ape, particularly gorillas, knot grasses to use as building materials in their nests.
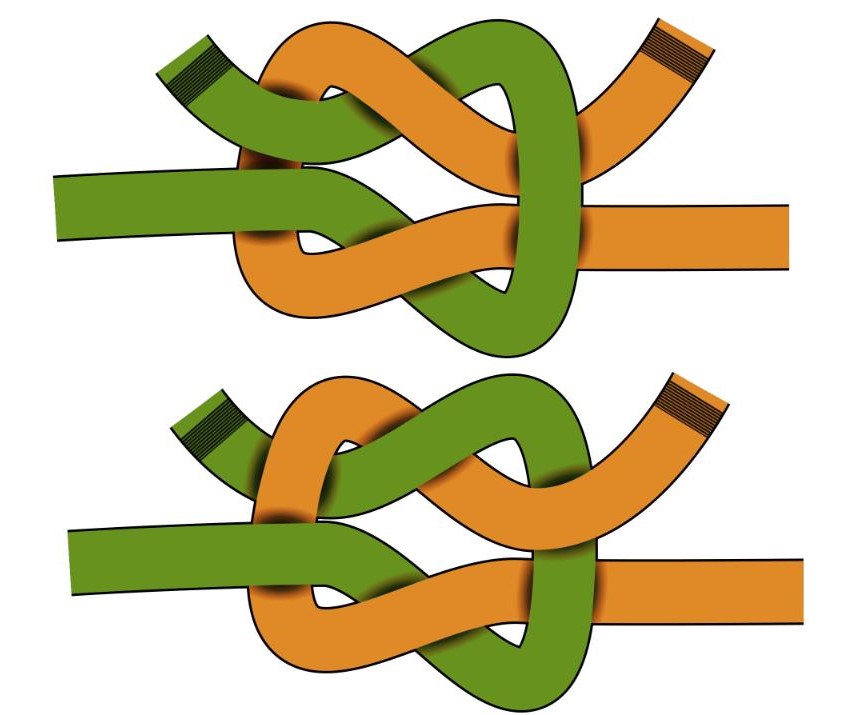
Despite their pervasiveness, experts have not yet cracked the code on many riddles associated with knots. When tying shoes, for instance, the granny knot looks a lot like the reef knot (or square knot), which is less common. Despite the fact that experience and tests have shown that the square knot is considerably more resilient, there has been no straightforward theoretical explanation for it. Now, thanks to the work of MIT mathematician Vishal Patil and his colleagues, that’s no longer the case.
A square knot, also known as a reef knot, is used for joining two ropes of similar diameter. It’s commonly used in various everyday applications, such as tying shoelaces, but it’s not recommended for critical loads because it can slip.
In a report published in the journal Science at the beginning of 2020, the researchers detailed three simple features that may be used to evaluate the stability of frequent knots. Patil’s group ran simulations and conducted tests to back up their theoretical findings. By doing this, they discovered a straightforward justification for the disparity in the reliability of various knots.
Though knots have been around for thousands of years, it wasn’t until recently that scientists began to study them properly. The “Iatrikon Synagogus,” a medical book published in the 4th century AD, has the first known textual description of several types of knots. Although knots were mentioned in that manuscript, they did not reappear in scientific writing until the 18th century. The widespread presence of mariners at the time meant that word quickly spread about the best implementations.
Mathematicians’ curiosity was piqued as a result of this as well. In 1794, while researching electromagnetism, Carl Friedrich Gauss was one of the first to attempt to define the many shapes that the field lines may take. Yet it was mostly his pupils who made significant contributions to the discipline. In order to analyze knots, they flattened the three-dimensional objects, generating a picture of intertwined curves.
Depending on the plane of projection used, the same knot might have many distinct two-dimensional representations. Because of this, it is possible for two seemingly dissimilar diagrams to represent the same thing. The question then arose among mathematicians as to what conditions lead to the equality of two knots diagrams. The group set out to categorize every potential knot, beginning with the simplest and working up to the most complex structures feasible.
Popular fishing knots include the Palomar knot, the Improved Clinch knot, and the Trilene knot. These knots are designed to provide high tensile strength for securing hooks and lures to fishing lines.

Knowledge of the unique characteristics of mathematical knots is essential for unraveling the mystery of this problem’s intricacy. To join two ropes, for instance, you would need to take two separate cords and tighten them around each other many times. As opposed to open knots, mathematical knots always consist of a single thread that is looped back on itself.
Topology is the cause behind this phenomenon. This branch of abstract mathematics is concerned with categorizing things based on their rough characteristics. If you can bend two figures into each other without creating any gaps or holes, then they are considered identical by topologists. The handle of a cup can be transformed into the center of a doughnut, rendering the two objects topologically identical.
Similarly, knots can be investigated using topological methods. One knot is equivalent to another if you can transform it into another by pulling and loosening it without severing the thread. A knot is always distinguishable from a “non-knot” if there are open ends, as in the case of shoelaces. Therefore, no knots with open ends exist from a topological perspective.
Mathematicians first concentrated on “real” knots, which have nothing to do with those in our daily lives. To categorize them, they proceeded as is common in topology: They were on the lookout for “invariants,” or characteristics of an object that would remain constant under the permitted deformations. The number of holes is an example of a topological invariant for two-dimensional surfaces, as shown by the cup and the donut. However, finding these invariants in knots is a difficult problem.
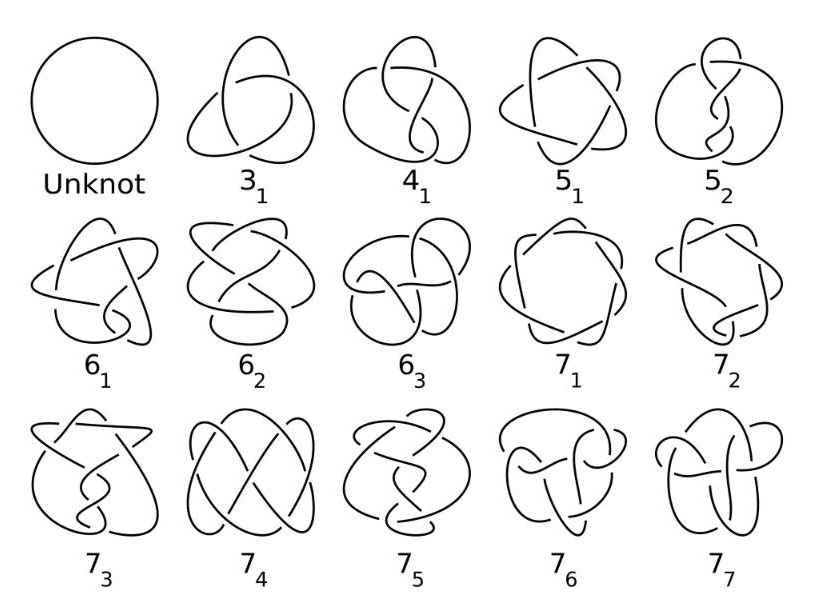
It is apparent to categorize knots according to the amount of crossed threads. However, counting “crossings” in a diagram is notoriously tricky. This is due to the fact that a knot’s number of vertices may vary depending on how it is projected onto a flat surface.
Climbers often use knots like the Figure-Eight Follow-Through and the Bowline because they offer a strong and secure connection when tying into harnesses or attaching ropes to anchor points.
No matter the projection plane you choose, there will always be at least one intersection at every mathematical knot. However, finding the representation with the fewest crossings is notoriously difficult when dealing with complex things.
Physicists and mathematicians have long since established that no knots exist that are connected by just a single or two intersections. But there is a class of knots that has three and four crossings in common, two that have five, three that have six, seven that have seven crossings, and so on. There is an exponential increase in the variety of knots present; for instance, 1,388,705 knots with 16 crossings can be found. It is not apparent, however, where this chain of events goes from here. Mathematicians have a long way to go before they can properly classify every possible knot.
Therefore, towards the middle of the 20th century, they created new techniques to handle the issue. One of the first to suggest disentangling knots in order to understand their constituent parts was the English mathematician John Horton Conway. The parts that fascinated him had four loose ends that were knotted together like the ones we’re used to seeing. These objects are called “braids” in technical jargon. Sailer and climbing knots are technically not knots but rather a braid of two strands.
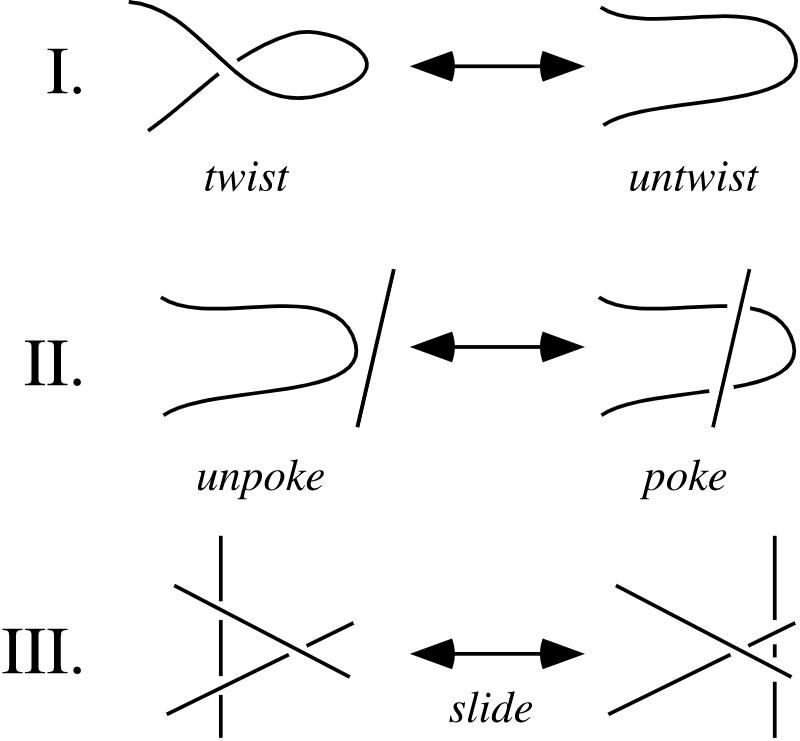
Three “Riedemeister moves” that do not alter a certain pigtail structure can be discovered using topology. As far as the stability of a knot goes (pigtail), this is not strictly true outside of the realm of pure mathematics. The first Reidemeister move, for instance, causes a strand to become tense.
Therefore, it is not sufficient to look at only topological considerations for deciding whether or not a knot employed in sailing is stable. Mechanical and elasticity theorems are also relevant to this discussion. This opens the door to a How, however, can one do so without being bogged down in mind-boggling math.


To bridge the gap between the reduced topological knot diagrams and the rough mechanical information, Patil and his colleagues developed a new notation. Their research focused on the challenge of tying a knot in two ropes to create a single, longer rope. That’s right; a two-strand braid has four free ends when analyzed statistically. They were interested in whether a knot can be made tighter by pulling on two of the loose ends, and if so, to what extent. That’s why they needed to find out what makes a tight knot so stable.
At every point where two strands cross, friction is formed and a rolling motion is initiated. A strand may roll away if its twists are all facing the same direction at each crossing. However, if the rotation directions are different, the system is stable, and the knot is harder to untie. Therefore, Patil and his coworkers developed a simple method to compare and contrast the various twists of a knot diagram, yielding the parameter “τ”.
The greater the value of τ, the greater the proportion of counterclockwise twisting motions, and hence the greater the stability of the knot. This characteristic allowed the researchers to determine the primary difference between a cross knot and a granny knot: The latter is less difficult to release since the direction of rotation is consistent. As a result, shoelaces tend to unravel easily.
However, the stability of some of the most popular knots can’t be told apart by just looking at τ. The thief knot is illustrative of this concept because, like the cross knot, it involves pulling on two strands of rope simultaneously but in opposite directions. When pulled, the twist in both knots is the same, but the thief knot can be untied more quickly.
As a result, Patil and his group investigated other pillars of support. It turns out that when the two adjacent strands of a knot are moved in opposite directions, the knot holds better. In addition, the researchers established a second variable, denoted by Γ, which is a count of how many strand portions pull together in opposing directions. The square knot, for instance, contains four such divisions, whereas the thief knot has just one. For this reason, the slip knot is more secure.
Researchers evaluated the stability of several knot families by counting the number of crossings (N), calculating the twists (τ), and counting the opposing strand sections (Γ). For this, they did not require expensive computer simulations or thorough computations, but only tallied qualities that could be readily derived from a knot diagram.
Without verification, none of these factors matter much. Because of this, Patil and his coworkers tried out some experiments with ropes that could change colors. These are made up of a core of elastic material surrounded by many layers of transparent elastomers with varying refractive indices. When the rope is bent or pushed, the elastomers thicken and change color. Therefore, a force map of a knot’s environment is now conceivable.
The team used this method to study simple knots, comparing the findings to those of related computer simulations and their theoretical model. The outcomes from the three approaches were identical. Next, they ran simulations of knots with more complex geometry, including the Zeppelin knot, which has 10 crossings, or the butterfly knot, which has twelve. The three variables (N, τ, Γ) imply that the butterfly knot, a popular knot among climbers, is less secure than the Zeppelin knot. This conclusion was also supported by computer simulations.